The 2024 SAT standardized test is a critical assessment for college admissions. The math section covers a wide range of topics. To help students prepare, we’ve created a practice paper. This paper includes various math problems to help students become familiar with the test format and excel in their exam.
1. Which of the following is a possible equation for a circle that is tangent to both the x-axis and the line x = 4 ?
A. (x + 2)² + (y + 2)² = 4
B. (x + 2)² + (y – 2)² = 4
C. (x – 2)² + (y + 4)² = 4
D. (x – 6)² + (y – 2)² = 4
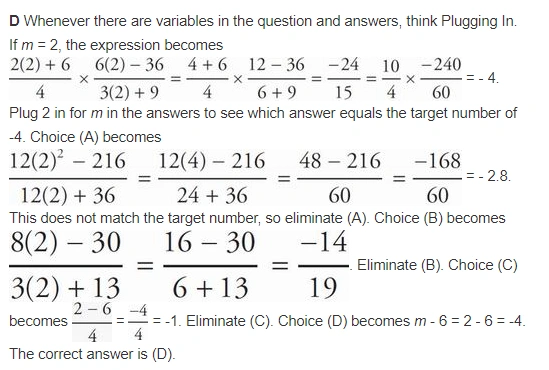
2. Reactant A is placed in a beaker, to which Reactant B will be added. Reactants A and B will not react unless B gets to a certain concentration. Once the reaction starts, both concentrations decrease until B has been consumed. Which of the following graphs, showing concentration in moles as a function of time in seconds, represents the reaction?
3.
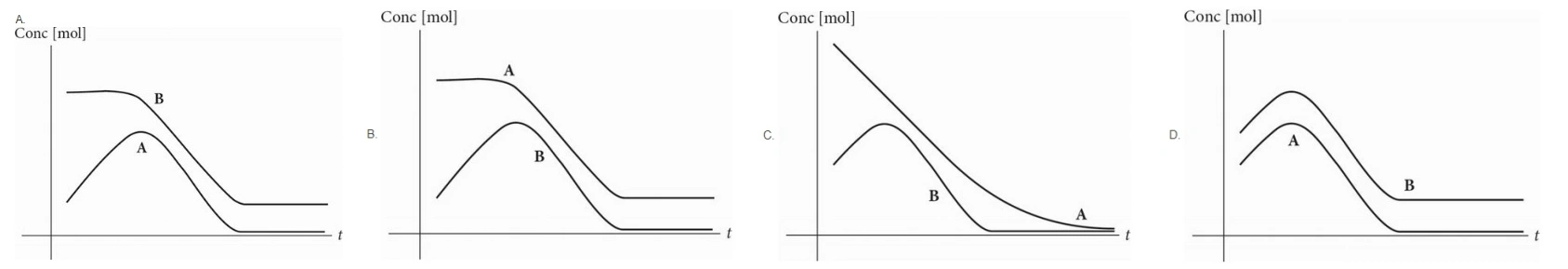
-2y ≤ 8
y – 3 ≤ x
-\frac{1}{3} y + 1 ≥ x
Which of the following graphs shows the solution to the system of inequalities above?
4.

If rectangle ABCD has an area of 324 and the tangent of ∠BCA (not shown) is \frac{4}{9} , then which of the following is closest to the length of BD (not shown)?
A. 9.8
B. 27
C. 29.5
D. It cannot be determined from the given information.
5. Which of the following is equivalent to \frac{2m+6}{4} x \frac{6m-36}{3m+9} ?
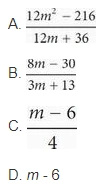
Warning: Undefined variable $in_same_cat in /www/wwwroot/mtexam.com/public_html/wp-content/plugins/EXP.GKFEED.COM/function.php on line 27
Warning: Undefined variable $excluded_categories in /www/wwwroot/mtexam.com/public_html/wp-content/plugins/EXP.GKFEED.COM/function.php on line 27