2024 SAT Standardized Test Math Practice Paper 12

The 2024 SAT standardized test is a critical assessment for college admissions. The math section covers a wide range of topics. To help students prepare, we’ve created a practice paper. This paper includes various math problems to help students become familiar with the test format and excel in their exam.

 

1. A developer is creating a plan for a 44-acre park that includes a 4-acre lake that cannot be developed. If 8 to 10 acres, inclusive, must be reserved for soccer fields, which of the following inequalities shows all possible values for p, the amount of land that within the park that is available for development?

A. 26 ≤ p ≤ 40
B. 30 ≤ p ≤ 32
C. 34 ≤ p ≤ 36
D. 36 ≤ p ≤ 40

Correct Answer: B

Answer Explanation:

In order to find the undeveloped area, take the entire area of the park and subtract the area of the developed portions. Subtract the 4 acre lake to get 44 – 4 = 40 undeveloped acres. Next, subtract the largest and smallest possible soccer field area: 40 – 10 = 30, and 40 – 8 = 32. Therefore, the correct answer is (B).

2. Environmentalists have been monitoring the area of a glacier in Canada. The glacier is slowly shrinking. The glacier originally occupied 15,000 square miles, but after two years of monitoring the glacier, the scientists document that the area of the glacier is now 14,910 square miles. If y is the number of years since monitoring began, which equation best describes the glacier’s area, G(y), as a function of time?

A. G(y) = 15,000​\( \frac{1}{y} \)​
B. G(y) = 15,000 \( (0.003)^y \)​y
C. G(y) = 15,000\( (0.997)^y \)​
D. G(y) = ​\( (0.997)^y \)​

Correct Answer: C

Answer Explanation:

Math 12 1

3. Mike consumes an average of 1,680 calories per day. Each day he has finals, Mike consumes 12% more calories per day than he usually does. During the last day of finals, he celebrates by consuming an additional 900 calories. Which of the following represents the total number of calories Mike consumes during d days of finals?

A. 1.12(1,680d + 900)
B. 1.12(1,680d) + 900
C. 1.12(1,680 + 900)d
D. (1,680 + 0.12d) + 900

Correct Answer: B

Answer Explanation:

First, calculate what Mike’s daily calorie consumption is during finals. 12% of 1,680 is 0.12 × 1,680 = 201.6. During finals Mike consumes 1,680 + 201.6 = 1,881.6 calories per day. Whenever the question includes variables, Plug in. Let d = 2. Over 2 days Mike consumes 2 × 1,881.6 = 3,763.2 calories. He also adds 900 calories at the end of finals. His total consumption over the entire finals period is 3,763.2 + 900 = 4,663.2 calories, so 4,663.2 is the target number. Plug in 2 for d in each of the answer choices. In (A), 1.12[1,680(2) + 900] = 4,771.2, which is not the target number. Eliminate (A). In (B), 1.12[1,680(2)] + 900 = 4,663.2, which is the target. Leave (B), but check the other answer choices just in case. In (C), 1.12(1,680 + 900)(2) = 5,779.2, and in (D), [1,680 + (0.12)(2)] + 900 = 2,580.24. Eliminate both (C) and (D). The correct answer is (B).

4. The varsity swim team at Northwest High is planning a team trip and needs to choose between Austin, TX, and  Pensacola, FL. The team takes a vote and the results of the vote are shown in the table below.

Math 12 2

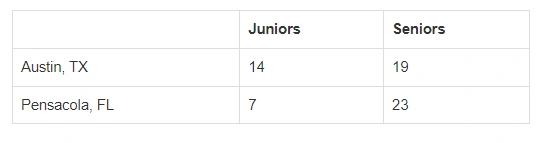
Given the information shown above, which of the following statements is true?

A. The number of juniors that prefer Pensacola, FL, is twice the number of juniors that prefer Austin, TX.
B. The seniors are more than three times as likely to prefer Pensacola, FL, than are the juniors.
C. The number of seniors that prefer Austin, TX, is 5% more than the number of juniors that prefer Austin.
D. One-third of the juniors prefer Pensacola, FL.

Correct Answer: D

Answer Explanation:

Use Process of Elimination on this question. Choice (A) cannot be correct because more juniors prefer Austin to Pensacola. Choice (B) sounds appealing, but “more than three times as likely” means the seniors as a whole need to prefer Pensacola more than three times as much as the juniors do as a whole. Seniors prefer Pensacola 23 out of 42, or 55%. Juniors prefer it 7 out of 21, or 33%. So, seniors do not prefer Pensacola more than three times as much as juniors do. You can also eliminate (C) because more than half of all juniors prefer Austin, while less than half of all seniors prefer Austin. The statement in (D) is correct since 7 is one-third of the total of 21 juniors.

5. The 2013 U.S. Census recorded the highest  educational attainment of all adults aged 25 years or older in county T, one of the most educated parts of the country. The results are given in the two-way table below.

Math 12 3

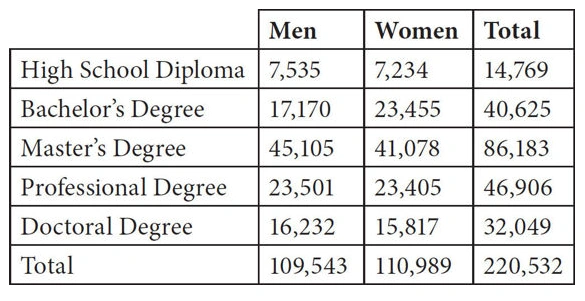
According to the data presented in the table above, if you were to choose a person at random out of the entire population aged 25 years or older in county T, what is the approximate probability that the person you chose is a man with a doctoral degree (given as a percent)?

A. 2%
B. 7%
C. 28%
D. 51%

Correct Answer: B

Answer Explanation:

We are looking for the probability that a randomly selected person is a man with a doctoral degree. There are 16,232 men with doctoral degrees, and 220,532 total adults aged 25 years or older. So the probability that a randomly selected person fits the category we are looking for is ​\( \frac{16,232}{220,532} \)​= 0.07 = 7%, which is (B).

Pages: 1 2

Warning: Undefined variable $in_same_cat in /www/wwwroot/mtexam.com/public_html/wp-content/plugins/EXP.GKFEED.COM/function.php on line 27

Warning: Undefined variable $excluded_categories in /www/wwwroot/mtexam.com/public_html/wp-content/plugins/EXP.GKFEED.COM/function.php on line 27
< PREVIOUS POST2024 SAT Standardized Test Math Practice Paper 11
NEXT POST >2024 SAT Standardized Test Math Practice Paper 13

Leave A Comment?